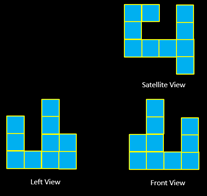
Effective teaching of mathematics

Effective classrooms help develop students’ mathematical and statistical identities and proficiencies by affirming their cultural identities, goals, and interests and incorporating relevant cultural contexts into teaching and learning programmes.

Guidance for effective practice:

Use contexts that are of genuine cultural significance to your ākonga (students) and that provide opportunities for mathematical challenge

Maia (teacher) works with her students on simple ratios to develop their multiplicative thinking. She uses the Samoan tradition of a to’ona’i, or Sunday lunch, usually held after church. The class discusses the family traditions from different cultures for sharing food in whānau, or wider groups. Her students work in collaborative teams to work out quantities of different foods they will need, such as sapasui (traditional Samoan chow mein).

• Maia: How much of each food is needed if we have a to’ona’i for our whole class?

Convey high and realistic expectations to all ākonga and encourage them to develop a "growth mindset" that all learning is achievable with effort

Emma starts every year with her students by developing a culture of personal agency. She praises effort over result, encourages students to ask questions and take risks, rewards persistence and flexibility in the face of challenge, and invites students to ask for feedback from her and from their classmates.

To encourage students to reflect on their learning, Emma asks students to write an exit ticket for some mathematics lessons. The tickets include prompts like:

• I love a good challenge. Today my challenge was...
• I know perseverance is important. Today I kept going when...
• My brain is growing all the time. Today it grew the idea...

Emma takes time to read the tickets in class during independent learning time, so she can talk to a few individual students. The tickets are glued into mathematics books and are used to inform parent interviews.

Use class routines that acknowledge the ways your ākonga prefers to learn and that enable efficient time management of tasks and discussions

Ken uses karakia at important transitions during the school day. An individual student shares their own message about important aspects to attend to, such as:

• Let’s keep the waka moving in the right direction. Listen to everyone in our crew. Give us strength to use our maths hoe (oars) so we can complete our mahi.

Students in Ken’s class express a desire to work together, so he devotes significant time to working in collaborative teams. Ken usually allows his students to choose their own team members.

An effective mathematics and statistics programme includes sustained daily engagement and opportunities to work both independently and collaboratively to make sense of ideas.

Through productive communication with whānau and by noticing what their students believe, think, and do, effective teachers of mathematics provide responsive learning experiences that enable students to build in their existing proficiencies, interests, and experiences.

Guidance for effective practice:

Acknowledge and build mathematics teaching upon the ideas of ākonga and their identities, cultures, and lived experiences

Tamsin has a year 7 class. She begins a unit of work on Movement and Position by asking students about how people in their whānau find their way to places they have not been to before.

Her students offer many scenarios of navigation, such as using the mobile phone (GPS), or a tool like Navman or TomTom, reading a map, using landmarks like the Skytower or a maunga, or asking a person for directions. One student retells the story of having to phone a relative to find out how to get to their place. The discussion is rich.

• Tamsin: We certainly have a lot of help to find our way nowadays. How did our ancestors find their way to Aotearoa/New Zealand in the first place?

Students suggest ways that Polynesian and European explorers may have found their way, including using the stars, and sailing around until they found land.

• Tamsin: Let’s watch a short television programme about how Polynesian people first sailed to Aotearoa.

She plays a four-minute segment of Te mana o te moana: The Pacific Voyagers from Educational TV (47:10-51:20). The programme discusses how about 1300AD Polynesian explorers used the sun and stars, ocean currents, prevailing winds, and sea birds to locate land.

Her students are enthused and investigate different ways that people around the world find their way. They find out that most main roads in New Zealand follow historic Māori walking tracks and use rivers and passes through mountain ranges.

In doing so Tamsin builds on the lived experiences of her students and contextualises the theme of navigation in the histories of their ancestors. She openly values diverse ideas about movement and position (see Meaney, Trinick, & Fairhall, 2013, for more on culture being internal to curriculum).

Notice and act upon partial knowledge constructions and misconceptions of your ākonga

Mandy works with Tai, a year 2 student, on early place value. Tai creates bundles of ten iceblock sticks, using rubber bands. He has loose sticks, as well.

• Mandy: Can you please give me forty iceblock sticks?
• Tai counts in tens putting a bundle in Mandy’s hand each time, "ten, twenty, thirty, forty".
• Mandy: Thanks Tai. How many bundles did you give me? (keeping hand closed)
• Tai: Ahhh...
• Mandy: Take a look. How many bundles?
• Tai: Four

Mandy notices that Tai’s focus is on counting in tens, not on how many tens are in the "-ty" numbers (decades). He has a partial early conception of place value.

Mandy might try two other decade numbers and see if Tai can generalize how many tens are in any "-ty" number.

Scaffold learning using strategies such as promoting interest, focusing attention, varying tasks, providing tools, and creating manageable goals (Te ako poutama)

Chad’s year 7 students are constructing cube models to match plan views (top, front, side) as part of an integrated unit on nzmaths called Cubic conundrums.

He notices that some of his students start on a model, realise the model does not match the views, and pull it apart to start again.

He suggests to students that the problem of getting all three views correct in one go may be too hard.

• Chad: Choose one view to get right. Which view is easiest?

The students mostly opt for the satellite view and construct that correctly.

• Chad: Now try building onto your satellite view to get the front view correct as well.

The students obtain a correct front view and modify it to get the left view correct as well.

Chad scaffolds the students’ learning by supporting them to break a complex task into smaller sub-goals.

Effective teachers understand that the tasks and examples they select influence how students come to view, develop, use, and make sense of mathematics.

Guidance for effective practice:

Provide open-ended, low-floor, high-ceiling problems that are relevant for ākonga and provide opportunities for multiple levels of sophistication

Moana has a year 3 class in an urban area. Students have worked hard on learning the counting sequence of whole numbers to 120, forwards and backwards.

Moana finds this problem that she thinks has potential:

• Imagine three houses in a row. What might the letter box numbers be?
• Is there an easy way to add all three numbers?

She encourages students to work in pairs and to record their thinking.

Moana has some enabling prompts to support pairs of students in making initial progress on the task. After an appropriate time of struggle, she offers a prompt to some pairs.

• Use these number cards, 1–20, to make a diagram of the letterboxes on one street. What do you notice?
• What sets of three letterboxes can you find?

Other pairs make considerable progress by creating many correct examples, like 4 + 6 + 8 = 18 and 1 + 3 + 5 = 9. Moana has some prepared extending prompts to encourage further thinking. An example might be:

• Choose the easiest example. Make the three numbers with towers of cubes. Even the towers up so that they are the same height. Look at the towers. What do you notice?

Provide sufficient practice opportunities for ākonga to develop fluency with important knowledge and skills. Highlight to your ākonga that knowing is important

At the beginning of the school year, Aaron notices that many of his year 7 students do not know their basic multiplication and division facts. This lack of knowledge impacts their ability to understand fractions, decimals, and percentages.

Aaron enrols in his class on e-ako. All the students use the multiplication and division learning tool to identify the facts they know and do not know.

Each day for the first three weeks, Aaron takes micro-lessons on patterns among the basic facts using a Slavonic abacus. He provides daily learning opportunities on e-ako and other websites, as well as games to practise the facts. Students take flash cards home to learn.

A "Number Mountain" checker is glued to the back of each student’s book, and they highlight when each layer of facts has been learned.

Choose tasks that invite ākonga to form and test conjectures, pose problems themselves, explain and justify their ideas, and generalise their findings

Tiere’s year 8 class is interested in whakapapa (genealogy), sparked by Tīpuna (Grandparent) Day at school. They first explore the growth pattern of direct biological relatives (mothers and fathers), which produces powers of two.

• Tiere: Let’s think about whānau. I know we have families of different sizes and arrangements, but we’ll start small. If a family has only two tamariki, what gender might the tamariki be?

The students conclude that the children might be two tama (boys), two kōtiro (girls), or a mixture of one girl and one boy.

• Tiere: If we take a sample of 100 two-tamariki families, what will happen?
• Allen (student): About 33, 33, 33, but not exactly (meaning the ratio of boy-boys to girl-girls to girl-boys).
• Matiu (student): I read that more boys are born than girls. Does that change things?
• Tiere’s students offer many different conjectures and ideas.
• Tiere: Nice ideas, but are they true? I want you to investigate what the chances are that a two-tamariki family will contain one boy and one girl.

Students work on the problem in groups of three. A few groups attempt to research the answer online. Tiere challenges them to think for themselves.

Some groups note the genders of tamariki in two child families they know; some survey their classmates to find the genders of the first two tamariki born; and others try to develop a theory.

After a class discussion, many students question the prediction of equal likelihoods. Tiere sets up a simulation in which bags of cubes (two colours) are used to select male or female babies. The results lead to the belief that mixed-gender families are more likely. Tiere’s students want to know why this happens.

Tiere gives her students tools to investigate the conjecture that boy-girl families are more likely to have a single gender. The tools include tables to organise data, tree diagrams to find all possible outcomes, and expectations about reporting results. Her students investigate. They justify their thinking using the results of trials, the data they have about families they know, and theoretical models like tree diagrams and tables. Some extend the problem to consider whanau with more tamariki.

Take advantage of the possibilities afforded by digital technologies

Bella is working on a statistical investigation with her year 7 and 8 students. She is an advocate for the strategic use of digital technology and has these criteria for when it is appropriate to use it.

• Students are highly motivated to engage with the mathematics presented.
• Multiple representations display simultaneously and facilitate connections among those representations.
• Risk-taking and creativity are encouraged through experimentation without consequence.
• Information is portable and easily replicated, which facilitates communication and collaboration.
• Students are provided with immediate, responsive feedback that supports their learning.
• Mathematics beyond that which is accessible with other media is explored, e.g., operations on very large numbers, complex symmetrical design.

She spends the first two days of her unit developing her students’ question-posing. Most students can ask specific summary, comparison, and relationship questions. Bella also models sampling from Census at School, saving and importing the CSV file into CODAP, and the basic graphing functionality of CODAP.

• Bella: I would like you to investigate the data about students who are your age. Take some time to see what questions students were asked, so you can see what data is available. Then pose a specific question you would like to investigate using CODAP.

Her students show a lot of interest in the data that was gathered in 2019. They write sound questions, though most are summary questions like:

• What is the reaction time of New Zealand year 7 and 8 students?
• What fraction of New Zealand year 7 and 8 students have a cellphone?
   
• Bella: The Census at School data are multivariate. What does that mean?
• Titus (student): There was a lot of different information gathered about each student.
• Bella: I want you to take advantage of that. Ask questions that involve comparisons or relationships.

Students revise their questions, and Bella highlights the characteristics of each question type as students create them. Her favourite question is:

• Is there a relationship between year 7 and 8 students’ height and how long they sleep for?

Her students work in pairs, using CODAP to display the data in different ways to investigate their question.

Finally, Bella’s students use PowerPoint to create a report that documents their question, their method of investigation, their findings, and a conclusion that discusses the implications of the findings.

Effective teachers support students in creating connections between different ways of solving problems, between mathematical representations and topics, and between mathematics and everyday experiences.

Guidance for effective practice:

Use a single context in the first few lessons about a mathematical idea, explore variations in the context, and consider the effect of that variation

Esa begins her unit on division with a single problem:

• Some sisters are given 36 marbles to share equally. How many marbles does each sister get?

Students explore possible answers by varying the number of sisters, using materials if needed.

Esa’s students generalise that the more sisters there are, the smaller the number of marbles they each get. They record their answers with equations, for example, 36 ÷ 3 = 12, organised in a pattern. The next few lessons are spent exploring variations of the same problem. For example,

• If there are four sisters, how many marbles would give them an equal share with no left-overs?

and

• Some sisters share some marbles. Each sister gets eight marbles. How many marbles and sisters are there?

Esa starts with a single division problem, 36 ÷ [ ] = [ ], and explores the effect of changing the location of the unknown.

Pose problems in contexts that help ākonga connect mathematics to their daily lives

Bik knows that her students are excited about the Rugby World Cup to be held in France. The boys and girls are obsessed with rugby and play the game every lunchtime. She also wants to know if her students can solve problems that combine the four operations.

She starts the lesson with a fake headline:

"Wallabies 30; All Blacks 27"

"Boring, booting Aussies beat enterprising, careless All Blacks."

• Is that possible?
• How did each team score their points?

Bik uses a context that is of high interest to her students and that affords investigation of important mathematical ideas.

Help ākonga to attend to pattern and structure

Pattern is about consistency, something that always occurs. Structure is about organisation, the way the elements in a pattern relate, and why that occurs.

Shirvani has a year 1 class. She notices that, generally, her students find repeating patterns challenging. Many of her students lack routines in their lives and are beginning to find comfort in the predictable sequence of events in a school day.

Shirvani decides to integrate pattern and shape into a unit of work. As well as using everyday objects like leaves and stones to make patterns, she uses sets of attribute (logic) blocks that she finds in the resource room. Her students find the blocks appealing.

In one lesson, she starts by building these patterns on the mat, introducing them one after the other:

• Shirvani: What do you notice? Talk to another person first.

Her students name the colours and shapes, and she highlights important words like triangle and circle.

• Shirvani: If I wanted to carry on this picture on all the way out the door, pointing in the right direction, what shapes would I need?

Some students are unsure about what comes next. Shirvani supports them in reading the patterns, "Blue circle, yellow circle, blue circle,..." Together, they extend the pattern further using attribute blocks.

• Shirvani: Think hard. What is different about the two patterns, and what is the same?

Shirvani is hoping that her students will notice the A, B, A, B, A, B, structure of both patterns, and possibly identify the part (unit) that repeats in each case.

Effective teachers use a range of assessment practices to make students’ thinking visible and to support students’ learning

Assessment for learning is best described as a process by which assessment information is used by teachers to adjust their teaching strategies and by students to adjust their learning strategies. Assessment, teaching, and learning are inextricably linked, as each informs the others (from Principles of assessment for learning).

Guidance for effective practice:

Provide ākonga with regular "dollops" of feedback and feed forward

Shelley’s year 5 class is learning to create and use equivalent fractions.

Two students, Aroha and Lelea, create other names for one half by folding paper strips in a trial-and-error fashion. They write correct equations, such as 1/2 = 2/4 and 1/2 = 4/8.

Shelley notices what is happening and has this conversation:

• Shelley: You did a good job of finding equivalent fractions for one half and writing them down. Please explain what you did.
• Lelea: We made halves first, then folded in half and half again.
• Shelley: I wonder what would happen if you folded one half into three equal parts.

[Aroha and Lelea start to fold a strip of paper.]

• Shelley: Hold on a second. Can you think ahead without doing it?
• Aroha: That’s hard. There would be three pieces inside one half.
• Shelley: What will you call those three pieces in one half? Why?

Shelley gives feedback to students on what they have done (folding and recording equations). She signals clearly through her request for imaging that the girls next steps include anticipating the result of the folding. Later, she draws the girl’s attention to patterns in the equations.

1/2 = 4/8                   3/4 = 9/12                  2/5 = 4/10

• Shelley: These equations are all correct, but I want you to look at why they are correct. What patterns can you see in the equations?
• Lelea: 1 x 4 = 4 and 2 x 4 = 8
• Shelley: Does that happen in the other two equations?
• Aroha: Not really; the second pattern is multiplying by three, 3 x 3 = 9 and 4 x 3 = 12
• Shelley: Remember folding the strips? Why are the numerator and denominator multiplying by three?

Make a lot of small assessment decisions every day by questioning your ākonga about what they are thinking

During a lesson on area and perimeter, Mikala is roaming the room while her students work in small teams on a collaborative task. Her students have access to squared paper, square tiles, and rulers. Jo allows access to calculators for a couple of teams. Here is the problem that her students solve:

• Farmer Jo wants to create a new rectangular run for her loyal sheepdog, Rusk.

She reads that the run should have an area of 36 m², since Rusk is a medium-sized Border Collie.

• To order the netting, Jo needs to know the perimeter of the run.
• How long might the perimeter be? Find all the possibilities using whole numbers of metres.

Mikala spends time with each group to find out what the students are thinking. Here is one conversation:

• Hoani (student): We tried using five, and that didn’t work.
• Mikala: What do you mean by "didn’t work"? You need to explain.
• Simone (student): If you make the run five squares across, like this, you get a square left over. 5, 10, 15 (counting rows of an array made with tiles)... 20.
• Mikala: Could you have known that 36 wouldn't divide evenly by five before you started building the array?
• Hoani: I don’t think so. You just need to try it out.
• Mikala: What is always true about multiples of five, answers to your five times tables? (writes a vertical list)
• Angela (student): Oh, I see, they all end in zero or five.
• Simone: Oh, no wonder five didn’t work with 36.

From her questioning, Mikala notes that these students need to connect multiplication with area and develop better knowledge of factors and multiples.

Use the same assessment task for multiple purposes: to establish and report what ākonga have learned up to a point in time, to diagnose the areas where they need support, and to inform them about their own learning so they can self- and peer-assess their progress

Kea syndicate uses a variety of assessment methods to inform teaching and learning, including tests, rich assessment tasks, short interviews, and work samples. The team uses Progress and Achievement Tests (PAT) early in the school year, about the beginning of March. All students attempt the same test at their class level. Marking is done automatically on the NZCER site to save on teacher time.

The teachers in Kea use the PAT reports both summatively and formatively. Using the List Report, teachers convert the PATM scale scores to levels of the Mathematics and Statistics Curriculum. The level measurement for each student, such as 2A or 3P, provides teachers with important information to support "best fit" judgements needed for reporting to the Board of Trustees and to the Kāhui Ako.

To identify areas of strength and weakness, syndicate members look at the Item Report across all classes and for individual classes. First, teachers look for items that their students performed poorly on. Those items are looked at to get a sense of what was asked. Second, teachers look for items that indicate collective strength for most students. The team compiles a list of "hit zones", areas in which their students need targeted teaching. Teachers check the Item Report for their class to see which "hit zones" apply.

Kea teachers develop their long-term plan, informed by the needs identified through PAT and through their own observations of students.

Effective teachers facilitate classroom dialogue that is focused on mathematical argumentation.

Guidance for effective practice:

Employ, and encourage ākonga to employ, talk moves to orchestrate learning productive conversations in group settings

Caroline believes in the power of Talk Moves to empower students to interact effectively in collaborative groups. As a pre-service teacher, Caroline frequently participated in role-playing where Talk Moves were practiced. Caroline also ran a professional development session for her colleagues on Talk Moves using the module Introduction to Talk Moves in PLD 360.

To prompt her students, she displays posters of the main talk moves around the room, with examples:

• revoicing (repeating what another person said, possibly clarifying their language)
• providing wait time (given a respondent adequate time to construct their answer)
• adding on (building on the ideas of another person)
• explaining (clarifying the ideas of another person)
• comparing (comparing the different ideas of two or more people)
• applying (using someone else’s idea to solve a new problem)
• justifying (showing why an idea or strategy is correct)

Caroline knows that Talk Moves takes regular practice to become habits. In the first few weeks, she points out to students whenever she uses the moves herself and prompts her students when they are working in groups. During whole-class summaries at the end of lessons, she asks students for examples of how they used the moves.

She also provides a chart of question starters to help her students begin constructive responses to their classmates.

Source: Makar, Bakker, & Ben-Zvi (2015).

Set clear expectations about what constitutes a mathematical argument

Page’s year 3 class is investigating what happens when odd and even numbers are added and subtracted. They begin by deciding what is meant by odd and even numbers. Using tens frames, they decide which numbers of students can be organised into pairs with "no odd person left out". The tens frames for even numbers, 2, 4, 6, 8, 10, are grouped together, and the left-over frames are discussed.

Her students conclude that an odd number of students have "an odd person left over" after the pairing is done. The class investigates the whole numbers from 11 to 20 and correctly classifies the numbers as even or odd. Using a Hundreds board, the class highlights the even numbers and notices that the numbers exist in the columns. Other numbers greater than 20 are investigated to establish if the column pattern continues.

Page poses this problem:

• If I add two even numbers or two odd numbers, is the sum always even or odd? Why?

Students investigate the question in pairs using blank tens frames, counters, and cubes. They record their thoughts for the sharing session.

• Page: Charley and Rynan, what did you find out?
• Rynan: We tried 4 + 6, 2 + 10, and 8 + 6. The answers were 10, 12, and 14.
• Page: What kinds of numbers are 2, 4, 6, 8, and 10?
• Charley: They are all even, and the answers are even.
• Page: That’s interesting. Did other people find that?

Many of the pairs produced examples. Page lists the examples as "even sums" and "odd sums", referring to the answer.

• Page: What do you notice?
• Leonard: All the answers are even. You always get an even answer.
• Page: Could we get some odd answers? How?

Several pairs have odd answers because they added even numbers to odd numbers by mistake, e.g., 3 + 4 = 7, 6 + 5 = 11, 1 + 8 = 9. The page lists the equations in the Odd Sums column.

• Page: Now I want you to go back and figure out why these things always happen. Why is it that you get an even answer if both addends are odd? Why is it that you get an odd answer when you add an even number to an odd number?

Page is aware that noticing patterns in examples is a first step to generalization. However, she wants her students to provide a justification for why these patterns occur. She expects students to go beyond specific numbers to the structure of any even or odd number.

Effective teachers shape mathematical language by modelling appropriate terms and communicating their meaning in ways that students understand.

Guidance for effective practice:

Work with ākonga to connect mathematical symbols with the meaning of those symbols in spoken and written language

Viliami is aware that the language of mathematics is sometimes confusing for his students, especially those for whom English is a second language. He knows that confusion about words and symbols can also lead to misconceptions. He begins the unit on multiplication and division by asking his students about the meaning of three sentences displayed on the whiteboard:

• Five multiplied by six equals thirty.
• Siali has three times as many pencils as Repeka.
• Our class of 24 students is divided into groups of four.

Viliami underlines words and terms that he thinks might have other, non-mathematical meanings in real life. His students offer their ideas about the words.

• Ellie: Multiplied by is like "it gets bigger", right?
• Repeka: Times are about clocks, usually. I think it means Siali has more pencils than me.
• Tyrese: Groups are bands that play music or dance, I think. I belong to a pātē group that beats drums.

Vilami recognises that students’ existing meanings, such as multiplication makes bigger, may lead to over-generalisations. He also sees that everyday contexts, like Tyrese’s illustration of groups, may help students relate to the mathematical meaning. He asks his students to draw a diagram and write a mathematical equation for each sentence to show what the sentence means.

During the discussion that follows, Vilami explicitly draws students’ attention to the ×, ÷, and = symbols to negotiate their meaning. For example, several students have drawn "five multiplied by six" as six sets of five. Vilami records 6 x 5 = 30 and asks for the meaning of each symbol. Students decide that the x symbol means "of", as in six sets of five.

Collectively create and share a list of important mathematical terms with your ākonga and provide them with access to an age-appropriate mathematics dictionary, either in book or digital form

Andreas creates a list of important terms during the first lessons of a unit on symmetry with his year 6 class. The list is displayed on the mathematics wall along with student work. As a "stock take" of the students’ understanding of the ideas, he asks them to work in pairs to write or draw examples of each term (e.g., line of reflection, translation, point of rotation, etc.). Later, the class shares their illustrations and chooses the clearest examples to go on the wall display.

For terms that are uncertain, such as regular hexagon, students look up their dictionary and agree on the meanings, e.g., regular as having equal angles and side lengths, not the standard size or consistently occurring, as in real life.

Effective teachers select tools and representations of mathematical concepts that are transparent, meaningful, and functional for students. Tools and representations include mathematical words, symbols, stories or metaphors, pictures or diagrams such as graphs, physical materials, and technology.

Guidance for effective practice:

Use materials to support ākonga to represent and work with mathematical concepts and procedures

Some students in Joel’s year 1 class are learning to count, read, and say numbers up to 20. Their one-by-one counting is getting reliable. Joel wants them to understand that adding one more to a set results in the next number in the counting sequence; for example, if one is added to six objects, then there are seven objects.

• Joel gives each student a number strip and a collection of counters.
• Joel: Can you please count five red counters and hold them in your hand?

The students all count five counters.

• Joel: If you put the counters on the strip like this (one at a time, starting at one), what number will the last counter cover?

Some students seem to think the answer is obvious, but others need to place the counters to check.

Joel gives each student one counter of a different colour, and he asks,

• If you put that counter on your strip, how many counters will you have then?

All the students say, "six", realising that the 6th circle will be covered.

He gives his students two more examples: eight counters and one more, and 12 counters and one more. In the last example, Joel gets the students to turn over the strip so they cannot see the numbers (masking).

Next, students put the number strip behind them and solve other "one more" problems without it.

• Joel: Get ten red counters for me. Put them in your hand and close it. If I give you one more counter, how many will you have? If I give you one more again, how many counters will you have?
• Joel: What do you think happens if I take one counter away instead?
• Joel supports his students to visualise the result of actions on materials through the gradual withdrawal of those materials.

Develop the diagrammatic and symbolic literacy of your ākonga to represent and work with mathematical concepts and procedures

Mel downloads the teaching unit Getting partial to percentages from nzmaths. She uses the percentage download bar for computer files to represent percentage problems throughout the unit.

Mel uses the words rate, base, and amount in conjunction with the bar model and supports her students to use that vocabulary to explain their strategies.

Her students become adept at using the percentage bar to support their problem-solving across a variety of types, depending on whether the rate, base, or amount is unknown. For example, Ofa creates this diagram to find [ ]% x 24 = 18.

Effective teachers develop and use sound knowledge as a basis for initiating learning and responding to the mathematical needs of all their students.

The problem requires students to recreate the whole shape, given one quarter of it. There are already plenty of possible shapes that can be made. To open the problem further and move beyond quarters, Callum changes the problem to this:

• Imagine there are many species of fraction bugs, like the two-thirds bug, the three-fifths bug, and the five-eighths bug. Choose a shape from the set of pattern blocks. If that is what each bug leaves behind, what does the whole shape look like? Find as many answers as you can.

Callum expects that students will need to make sense of less common fractions to solve the problem. The demands of the problem will encourage students to connect fractions and compositions of shapes.

Anthony, G., & Walshaw, M. (2007). Effective Pedagogy in Pāngarau/Mathematics: Best Evidence Synthesis Iteration [BES]. Wellington, New Zealand: New Zealand Ministry of Education.

Anthony, G., & Walshaw, M. (2009). Effective pedagogy in Mathematics. Wellington, New Zealand: New Zealand Ministry of Education.

Bishop, R., & Berryman, M. (2009). The Te Kotahitanga effective teaching profile. Set: Research information for teachers, 2(2), 27–33.

Charalambous, C. Y., & Praetorius, A. K. (2018). Studying mathematics instruction through different lenses: Setting the ground for understanding instructional quality more comprehensively. ZDM, 50(3), 355–366.

Hattie, J., Fisher, D., Frey, N., Gojak, L. M., Moore, S. D., & Mellman, W. (2016). Visible learning for mathematics, grades K-12: What works best to optimize student learning. Corwin Press.

Hunter, R., Hunter, J., Jorgensen, R. & Choy, B. H. (2016). Innovative and powerful pedagogical practices in mathematics education. In K. Makar, S. Dole, M. Goos, J. Visnovska, A. Bennison, & K. Fry (Eds.), Research in Mathematics Education in Australasia 2012–2015 (pp. 213–234). Dordrecht, The Netherlands: Springer International Publishing.

Makar, K., Bakker, A., & Zvi, B. (2015). Scaffolding norms of argument-based inquiry in a primary school mathematics classroom. ZDM Mathematics Education, 2015 (47), 1107–1120.

Meaney, T., Trinick, T., & Fairhall, U. (2013). One Size Does NOT Fit All: Achieving Equity in Māori Mathematics Classrooms. Journal for Research in Mathematics Education, 44, 235–263. 10.5951/jresematheduc.44.1.0235.

Schoenfeld, A. H., Baldinger, E., Disston, J., Donovan, S., Dosalmas, A., Driskill, M.,... & Zarkh, A. (2020). Learning with and from TRU: Teacher educators and the teaching for robust understanding framework. In International Handbook of Mathematics Teacher Education: Volume 4 (pp. 271–304). Brill Sense.

Sullivan, P. (2011). Teaching Mathematics: Using research informed strategies. Melbourne, Australia: Australian Council for Educational Research.