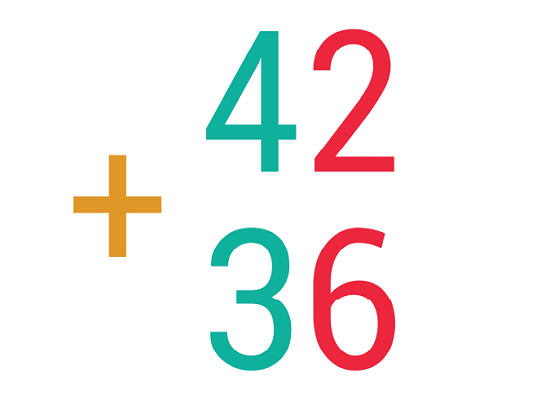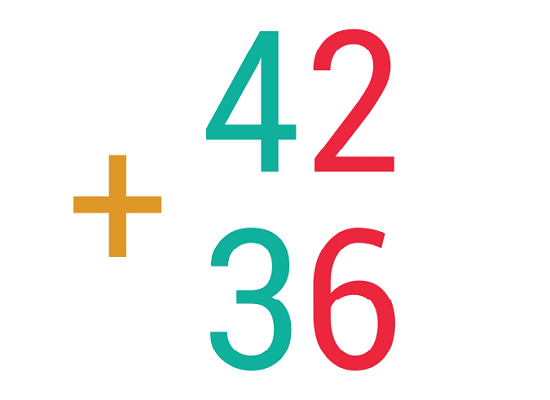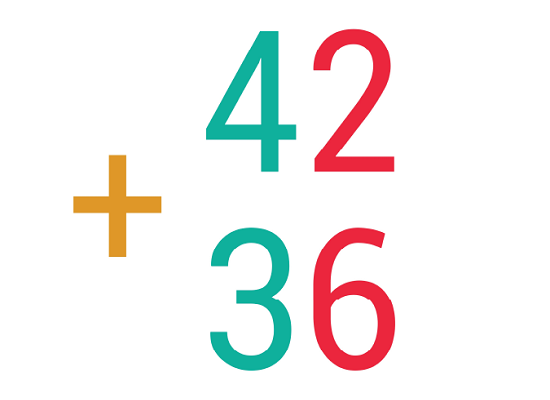Whole number place value for 2-digit addition & subtraction
This resource supports teachers to assess and find appropriate activities for students who need acceleration in their understanding and application of using whole number place value for two-digit addition and subtraction.

About this resource
New Zealand Curriculum: Level 2 to early Level 3
Learning Progression Frameworks: Additive thinking, Signpost 5 to Signpost 6
These activities are intended for students who understand place value with two-, and possibly three-digit numbers but are not yet able to use this knowledge to solve two-digit addition and subtraction problems. For some students, revision of addition and subtraction basic facts (number bonds to 20) may be needed prior to and alongside the use of these activities.
Whole number place value for two-digit addition and subtraction
The following diagnostic questions indicate students’ understanding of, and ability to use, place value to solve two-digit addition and subtraction problems. The questions are presented in order of complexity. If the student answers a question confidently and with understanding, proceed to the next question. If not, use the supporting activities to build and strengthen their fluency and understanding. Allow access to pencil and paper, but not to a calculator.
The questions should be presented orally and in written form (Diagnostic questions), so that the student can refer to them. The questions have been posed in a money context but can be changed to contexts that are engaging to your students.
Required materials
See Materials that come with this resource to download:
- Diagnostic questions (.pdf)
Activities
You have 60 dollars and I give you 50 dollars.
- How many dollars do you have now?
Signs of fluency and understanding
Using a part-whole strategy that applies place value, such as 5 + 6 = 11 so 50 + 60 = 110 or 50 + 50 = 100 so 50 + 60 = 110. The students show that they can work with tens as units in the same way they work with units of one.
What to notice if your student does not solve the problem fluently
Counting up in tens, 60, 70, 80, 90, 100, 110, may be a sign that the student does not understand that groups of ten and one hundred can be treated just like units of one; for example, 6 + 5 = 11, so 60 + 50 = 110, 600 + 500 = 1 100, etc. In this problem, counting may also be a sign that ‘tens within hundreds’ understanding is not established, that is, 11 tens equal 110.
Supporting activity
You have 22 dollars and I give you 35 dollars.
- How many dollars do you have now?
Signs of fluency and understanding
Using a place value-based strategy that adds the tens and ones separately then combines them to get the answer, such as 20 + 30 = 50 and 2 + 5 = 7, so 22 + 35 = 57. The student shows that they can work with tens as units in the same way they work with units of one.
What to notice if your student does not solve the problem fluently
Counting on in tens and ones may be another sign that the student does not understand that groups of ten and one hundred can be treated just like units of one. Counting strategies might be “22, 32, 42, 52, 53, 54, 55, 56, 57” or “35, 45, 55, 56, 57.”
Improvised part-whole strategies can also cause problems for students where there is an extra load on working memory. Look for signs like, “I started with 22. I took off the two and added 30 to get 50. Then I added the two back on ... , etc.”
Supporting activity
You have 79 dollars and spend 34 dollars.
- How many dollars do you have left?
Signs of fluency and understanding
Using a place value-based strategy that subtracts the tens and ones separately and then combines them to get the answer, such as 70 - 30 = 40 and 9 - 4 = 5 so 79 - 34 = 45. The student shows that they can work with tens as units in the same way they work with units of one.
If your student uses a written algorithm, ask them about the meaning of their working to check that they are applying place value knowledge. Lack of understanding shows when students think that all the digits refer to ones, e.g., “I carried the 1.”
What to notice if your student does not solve the problem fluently
Counting back in tens and ones may be another sign that the student does not understand that groups of ten can be treated just like units of one. Counting strategies might include “79, 69, 59, 49, 48, 47, 46, 45” or “79, 78, 76, 75, 65, 55, 45.”
Improvised part-whole strategies can also cause problems for students where there is an extra load on working memory. Look for signs like, “I took 9 off the 79 to make 70, then I took off 30 to get 40. I added the 9 back on, then took away 4 to get 45.”
Supporting activity
You have 47 dollars and I give you 38 dollars.
- How many dollars to you have?
Signs of fluency and understanding
Using a place value-based strategy that involves renaming ten ones as one ten, such as 47 + 30 = 78, 78 + 9 = 87 or 8 + 7 = 15, 40 + 30 = 70, 70 + 15 = 85
If your student uses a written algorithm, ask them about the meaning of their working to check that they are applying place value knowledge. Lack of understanding shows when students think that all the digits refer to ones.
What to notice if your student does not solve the problem fluently
Counting on is unlikely to occur since a student who prefers that strategy will not have proceeded to this problem. However, counting based strategies include “47, 57, 67, 77, 78, 79, 80, 81, 82, 83, 84, 85.”
Part-whole strategies, especially inefficient ones, can cause difficulties due to the load on the student’s working memory. A student may lose track of parts in their mental calculation. Look for signs like, “I took 7 off the 47 to make 40, then I added 30 to get 70. I added on the 8 to get 78” (forgetting the 7 from 47).
Supporting activity
Adding two-digit numbers with renaming
You have 83 dollars, and you spend 36 dollars.
- How many dollars do you have left?
Signs of fluency and understanding
Using a place value-based strategy that involves renaming one ten as ten ones or subtracting back through a decade, such as 83 - 30 = 53, 53 - 6 = 47 (going back through 50).
If your student uses a written algorithm, ask them about the meaning of their working to check that they are applying place value knowledge. Lack of understanding shows when students cannot explain how and why they changed the numbers.
What to notice if your student does not solve the problem fluently
Subtracting lower digits from higher digits is a common error. Look for strategies like “80 – 30 = 50, 6 – 3 = 3, so 83 – 36 = 53.
Inefficient strategies place a considerable load on working memory. A student may lose track of parts in their mental calculation. Look for signs like, “I took 3 off the 83 to make 80, then I subtracted 30 to get 50. I took away the 6 to get 44” (forgetting to add on the 3 from 83).
Supporting activity
Heading
The quality of the images on this page may vary depending on the device you are using.