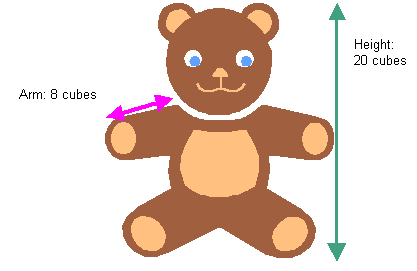
Teddy bears and friends
In this unit we compare the lengths of ākonga soft toys directly, and then indirectly using non-standard measurement units.

About this resource
Specific learning outcomes:
- Compare a group of 3 or more objects by length.
- Measure length with non-standard units.
Teddy Bears and Friends
Achievement objectives
GM1-1: Order and compare objects or events by length, area, volume and capacity, weight (mass), turn (angle), temperature, and time by direct comparison and/or counting whole numbers of units.
Description of mathematics
In this unit ākonga begin by making direct comparisons between objects and putting a number of objects into order according to length. They are also introduced to measuring with multi-link cubes which allows them to compare objects which cannot be placed together.
Multi-link cubes are an example of a non-standard measuring unit. They reinforce most of the principles that underpin measurement and allow ākonga to find out that:
- you must not change the unit being used when you are measuring an object.
- units are chosen for their convenience and appropriateness to the object being measured.
- units are placed end to end in a straight line and then counted to find the distance (length) between two points.
- you express measurements to the nearest whole unit or to a specified degree of accuracy, for example, almost 5 handspans, or about 6 ½ straws long.
Ākonga will also be encouraged to estimate. Initially these estimations may be little more than guesses, but estimating involves ākonga in developing a sense of the size of the unit. The skill of estimating is just as important as finding exact measurements, as both skills are used frequently in everyday life, for example, estimating shoe size before trying on a shoe, knowing exact height to go on a waterslide.
Opportunities for adaptation and differentiation
The learning opportunities in this unit can be differentiated by providing or removing support to ākonga and by varying the task requirements. Ways to support ākonga include:
- providing or removing help to ākonga to draw outlines
- supporting ākonga to make measurements using cubes. This provides an opportunity to support those who need help with the correct placement of cubes or help in counting the number of cubes needed
- providing some ākonga with other units of non-standard measurement they could use to measure (for example, finger length, lego, strips of paper). Some ākonga may wish to explore with a ruler (standard form of measurement) as well.
As the focus of this unit is making measurements of themselves it is already in a context that is meaningful. In some situations, it may be more appropriate to use a collection of classroom objects rather than ask students to bring toys to school.
The context for this unit can be adapted to suit the interests and experiences of your ākonga. For example:
- providing other objects to measure that your ākonga may enjoy, for example, shoe length, rocks from playground or lunchbox lengths
- ākonga could go beyond the classroom and measure other objects from around their kura and community, for example, playground equipment, parts of marae, gates and pathways.
Te reo Māori vocabulary terms such as ine (measure), roa (long), poto (short), nui (big) and iti (small) could be introduced in this unit and used throughout other mathematical learning.
Required Resource Materials
- multi-link cubes (or blocks)
- cuisenaire rods (the 10 rod is best)
- scissors
- a teddy bear or soft toy from home
- large sheets of paper for drawing around the toys (A3 or A2)
- a large roll of paper for drawing outlines of ākonga
Activity
1.
We begin the week by looking at all the soft toys ākonga have brought to kura. Ask the ākonga to introduce their soft toy to the class. The picture book There's a Bear in the Window by June Pitman-Hayes, and translated by Pānia Papa, could be used to ignite interest in this context.
2.
Ask a ākonga to put their toy in the centre of the circle.
- Who has a toy that is taller than this?
- Who has a toy that is shorter than this?
- Who has a toy that is the same height as this?
3.
Let ākonga take turns bringing their toys into the centre to compare. Ask ākonga to show you how they know the toys are taller, shorter, and the same height. Encourage students to identify that the starting point of the measurement must be the same when comparing the height of the toys.
4.
Put taller toys in one group, shorter toys in another and toys of the same height in the third group.
5.
After the heights have been compared, ask the ākonga to suggest other ways that the toys could be compared. For example: bigger or smaller feet, longer or shorter legs, bigger or smaller puku.
6.
Ask groups of 3 ākonga to put their toys into an order. As they do this, ask questions that require them to describe the size of the attribute (type) they are using as a referent. For example:
- What order have you put these toys in?
- Why is this toy placed here?
- Can you order them in a different way?
7.
See if other ākonga can guess the attribute that the groups have used to order their toys.
8.
Show ākonga how to trace outlines of their toys on paper which they can colour to make life-sized portraits for use later in the week.
For the next 3 days we make comparisons using ākonga. In pairs, ākonga take turns drawing outlines of their bodies. A tuakana/teina model could work well here. They use these outlines to make measurements using multi-link cubes or cuisenaire rods (the 10 ones work best). Kaiako or ākonga can record their estimates and actual measurements as appropriate.
1.
Demonstrate how to draw around an ākonga to get an outline. Show that they need to draw around both arms and legs. This could be done with chalk outside if it is a fine day.
2.
Give each of ākonga a cube and ask them to estimate (guess) how many cubes you would need to measure the length of the arm.
3.
Check the estimates by measuring with the cubes.
4.
Now give them a cuisenaire rod and ask them to estimate again. By asking them to explain or justify their guess, you can focus their attention on the size of the rod in comparison to the cube.
5.
Check the estimates by measuring with the rods.
6.
Ask ākonga what other parts of the body they would like to measure. List these on a chart (with drawing) for later reference. Be wary of students' feelings about their bodies.
7.
Ākonga can then go back to their pairs and outlines and measure the length of their legs, feet, fingers (for example) using either cubes or rods.
8.
If ākonga choose to measure their waist you will need to discuss how they can measure around something. Discuss how they could use string to mark off the distance around their waist and then measure the string with cubes.
9.
Ask ākonga to record their measurements on their outline.
10.
Once ākonga complete measuring their outline, ask them to measure the outline of their toy.
11.
At the end of each day, share mahi and make comparisons. Remember to make comparisons amongst the same type (toys or ākonga, in this case).
- Whose arm measured more than 25 cubes?
- How many more?
- Which parts of your body were measured shorter than your arm?
- Which is your smallest measurement?
- Which is your largest measurement?
- What have you measured with rods? Why did you choose rods?
- Have you ever been to a place where you were measured? Tell us about it.
Today we line up the outlines of our soft toys ready to go to kura assembly with (the shortest in the front.)
1.
Ask ākonga to measure the height of their soft toy using multi-link cubes and record this on the outline. Have them cut off any extra paper from the top and bottom of the outline.
2.
Tell ākonga that the toys want to go to the kura assembly, and so that everyone can see them, they will need to stand in order from the short ones to the tall ones.
3.
Ask four ākonga to put their toy outlines in order. This could be done using a line on the floor/corridor or the edge of the whiteboard. Let other ākonga check the order.
4.
Other ākonga can now put their toy outlines in the line. As they place their toy outline in the line, ask them why they have chosen that place.
5.
Continue discussing, comparing and moving outlines until all the toys have been ordered.
6.
Display the line in the hallway so that other ākonga and whānau can see it.
Home link
Dear family and whānau,
This week at kura we have compared the lengths of different objects including the toys you brought to kura. We would like your child to have a go at ordering their other toys at home, in order of shortest to tallest. Cutlery, food items or clothing could be used instead of toys. Take a photo or draw a picture of your line up and send it to kura for your tamariki to share.
The quality of the images on this page may vary depending on the device you are using.