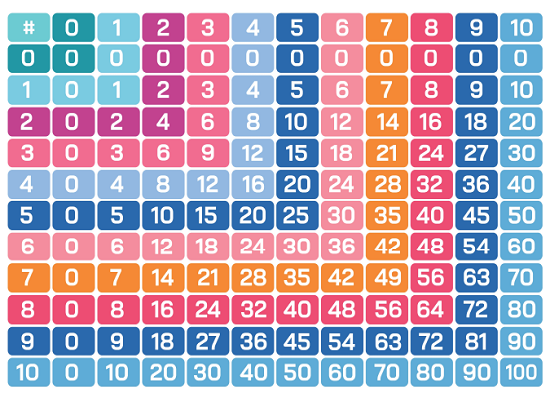
Multiplication as a binary operation

Lay down five cards, with three cars on each card. Arrange the cards in an array so the cars appear as they would in a car park.

• The cars are in rows of three. (Point along a row.) There are five rows.

Cover the cars with a masking sheet.

• How many cars are in the car park?

Signs of fluency and understanding

• Anticipates that 15 cars are in the carpark using a known fact, such as 5 x 3 =15.
• Anticipates that 15 cars are in the carpark by deriving from a known fact, such as 5 x 2 =10, 10 + 5 = 15. This strategy may be due to not knowing 5 x 3 =15.

What to notice if your student does not solve the problem fluently

• Counting by ones, often by pointing one at a time at imaged cars, indicates a lack of understanding that numbers can be treated as composites (sets).
• Skip counting, such as 3, 6, 9, 12, 15, indicates a dependence on counting in sets and an inability to form factors and apply multiplication. Note that students may see an opportunity to “count in threes” but lack the knowledge of sequence to enact the strategy.
• Repeated addition, such as 3 + 3 = 6, 6 + 3 = 9, 9 + 3 = 12, 12 = 3 = 15, indicates a dependence on addition and an inability to form factors and apply multiplication.

Supporting activities

• Forming factors

Lay down eight cards with ten cars on each card. Arrange the cards in an array so the cars appear as they would in a car park.

• The cars are in rows of ten. (Point along a row.) There are eight rows.

Cover the cars with a masking sheet.

• How many cars are in the car park?

Signs of fluency and understanding

• Uses a known fact, such as 8 x 10 = 80 or 10 x 8 = 80.

What to notice if your student does not solve the problem fluently

• Skip counting, such as 10, 20, 30, …, 80, or 5, 10, 15, …, 80, indicates a dependence on counting in sets (skip counting) and an inability to form factors and apply multiplication. Note that students may lack place value knowledge (that the 8 tens equal 80) and may therefore use skip counting as a trusted strategy.
• Repeated addition, such as 10 + 10 = 20, 20 + 20 = 40, 40 + 40 = 80, indicates a dependence on repeated addition and an inability to form factors and apply multiplication. This strategy does suggest ability to double and control the number of tens.

Supporting activities

• Selecting multiplication facts

Lay down two cards with six cars on each card. Arrange the cards in an array so the cars appear as they would in a car park.

• The cars are in rows of six. (Point along a row.) There are two rows.

Cover the cars with a masking sheet.

• What multiplication fact tells me how many cars are in the car park?
• How many cars is that?

Take away the masking sheet and double the number of rows (four rows of six).

• I am putting two more rows of cars in the car park (putting on two extra rows of six, then re-shielding the car park).
• What multiplication fact tells me how many cars there are now?
• How many cars is that?

Repeat the doubling to form eight rows of six and ask the same questions.

Signs of fluency and understanding

• Forms the correct multiplication facts for each step: 2 x 6 = 12, 4 x 6 = 24, 8 x 6 = 48. This indicates that the student knows the correct facts.

What to notice if your student does not solve the problem fluently

• Forms the correct multiplication facts for each step: 2 x 6 =, 4 x 6 =, 8 x 6 =. Knows that 2 x 6 = 12, but may find the other answers by addition, such as 12 + 12 = 24, 24 + 24 = 48. This strategy indicates knowledge of forming factors and the effect of doubling. It also suggests that the required basic facts are unknown.
• Forms the correct multiplication facts for each step but returns to establishing the total number each time, possibly by addition or skip counting. Sees no opportunity to build on the previous result. This strategy suggests an inability to build on known facts by doubling.

Supporting activity

• Deriving facts by doubling

Lay down four cards, with six cars on each card. Arrange the cards in an array so the cars appear as they would in a car park.

• The cars are in rows of six. (Point along a row.) There are four rows.

Cover the cars with a masking sheet.

• What multiplication fact tells me how many cars are in the car park?

Record 4 x 6 on a card, regardless of whether the student gives the fact. Place the card on top of the masked collection.

Repeat the steps with eight cards of three cars (8 x 3).

• Which car park has more cars? How do you know?

Signs of fluency and understanding

• Recognises that both carparks hold the same number of cars by doubling and halving the factors. “If you cut each row of six in half, you get a row of three cars. You need twice as many rows to have the same number of cars.”

What to notice if your student does not solve the problem fluently

• Calculates the number of cars in each car park using 4 x 6 = 24 and 8 x 3 = 24. States that both answers are the same but cannot explain why. This strategy may indicate that the student is unaware of doubling and halving as a strategy to derive new facts.
• Improvises from known facts to find the total number of cars in each park, such as 4 x 5 = 20, so 4 x 6 = 24. Focuses on getting answers and does not recognise the doubling and halving of factors. This strategy may indicate that the student is aware of some ways to derive multiplication facts.

Supporting activity

• Doubling and halving to find factors

Lay down eight cards, with five cars on each card. Arrange the cards in an array so the cars appear as they would in a car park.

• The cars are in rows of five. (Point along a row.) There are eight rows.

Cover the cars with a masking sheet.

• What multiplication fact tells me how many cars are in the car park?
• I am putting two more cars on each row (putting an extra two cars on each row, shielded from the student).
• What multiplication fact tells me how many cars there are now?
• How many cars are parked now?

Signs of fluency and understanding

• Knows 8 x 5 = 40 and 8 x 7 = 56 fluently and does not need to derive one fact from another. Ask, "Why is 8 x 7 = 56, 16 more cars than 8 x 5 = 40?"
• Deriving from a known fact, 8 x 5 = 40, to work out a related fact, 8 x 7 = 56, by adding 8 x 2 = 16. This strategy suggests that the student understands the distributive property in this example through 8 x 7 = 8 x 5 + 8 x 2.

What to notice if your student does not solve the problem fluently

• Calculates the number of cars separately for each situation. The student may do so using repeated addition. This strategy may indicate that the student does not understand or elect to use the distributive property.

Supporting activity

• Using the distributive property