Difference with renaming in two places
The purpose of this activity is to support students in solving subtraction problems with three-digit numbers that involve decomposing and renaming one ten as ten ones and one hundred as ten tens. Fluency in these strategies is important, as inefficient strategy use with these difficult subtractions can result in memory overload.
About this resource
New Zealand Curriculum: Level 2 to Level 3
Learning Progression Frameworks: Additive thinking, Signpost 6 to Signpost 7
These activities are intended for students who understand place value with two- and three-digit numbers but are not yet able to use this knowledge to solve addition and subtraction problems. While these activities assume students know most of their addition and subtraction basic facts (number bonds to 20), some students may need support to revise or develop these. For students who are not yet able to use place value to solve two-digit addition and subtraction problems, see Whole number place value for 2-digit addition & subtraction.
Difference with renaming in two places
Achievement objectives
NA3-1: Use a range of additive and simple multiplicative strategies with whole numbers, fractions, decimals, and percentages.
Required materials
- scissors
- calculators
See Materials that come with this resource to download:
- Place value people 1 (.pdf)
- Place value to 4 digits 2 (.pdf)
1.
Pose simple problems to establish the concept of difference and to discuss how to solve difference problems. Use, or alter, the context from the diagnostic question.
- There are 70 students at Rere School and 30 students at Kopu School. How many more students are at Rere School than Kopu School?
2.
Model the problem with Place value people 1 on a Place value to 4 digits 2.

3.
Discuss:
- How many more students does Kopu School need to have the same number as Rere School?
- What operation can we use to work that out?
Students are likely to suggest the most obvious strategy: adding on 30 to make 70. Record this operation using an empty number line and equation.

4.
Ask students if there is a way these numbers could be rearranged to form another expression with the same meaning (e.g., what is the difference between 30 and 70?). Support them to identify 70 - ? = 30 as a suitable equation. Emphasise that we know this because 30, 40, and 70 belong to the same family of addition and subtraction facts.
5.
Ask students if there is a way that 40 could be added to the subtraction equation while maintaining an unknown quantity. Support them to identify 70 - 40 as a suitable expression and represent this using an empty number line and an equation.

6.
Have students use place value people to model all three expressions discussed (30 + ? = 70; 70 - ? = 30; 70 - 40 =?). They might identify 40 + ? = 70 as an additional expression that shows the difference between 30 and 70 and represent this using their materials.
7.
Provide further easy difference examples to illustrate how the difference can be found with addition or subtraction. Consider your students' basic addition and subtraction facts knowledge when posing these questions, and avoid posing questions that will add additional memory load (e.g., questions that require students to work back or forth through 100s or 10s). For example:
- There are 400 students at Rere School and 100 students at Kopu School. How many more students are at Rere School than Kopu School?
- There are 650 students at Rere School and 300 students at Kopu School. How many more students are at Rere School than Kopu School?
8.
Provide an example where subtraction is more efficient than adding on and does not involve renaming. For example:
- There are 999 students at Rere School and 333 students at Kopu School. How many more students are at Rere School than Kopu School?
Encourage students to use the Place-value-people-1, as needed, while moving them towards the use of their symbolic recording methods (e.g., empty number lines, equations, vertical written algorithms).
9.
Share the strategies that students chose. You might have students share with a partner or a small group (perhaps completing a thinking map with their peers) before providing time for students to share with the wider class. Use a calculator to confirm the answer.
- What operation do I enter into my calculator?
Note that 333 + ? = 999 is not possible on a four-function calculator. (More advanced CAS calculators allow that entry).
999 – 333 = 666 is the viable operation to find the difference.
10.
Ask students to consider which strategies are more or less efficient. Look for students to identify adding on using an empty number line as one example of a strategy that uses many steps, therefore making it less efficient. Using a vertical written algorithm, which involves fewer steps (and no renaming), is "more efficient".
If your students are unfamiliar with the use of vertical written algorithms for subtraction, you might use this time to model the connection between a three-column place value representation of 999 - 333 (or perhaps 99 - 33 to start with) and an algorithmic representation.

11.
Pose a three-digit difference (subtraction) problem that requires renaming. Let students solve the problem in any way they like. Provide materials and supports as needed (e.g., grouped work opportunities, worked examples, calculators to confirm solutions). For example:
- There are 806 students at Rere School and 649 students at Kopu School. How many more students are at Rere School than Kopu School?
12.
Share the strategies that students chose. You might have students share with a partner or a small group (perhaps completing a thinking map with their peers) before providing time for students to share with the wider class. Use a calculator to confirm the answer.
13.
Ask students to consider which strategies are more or less efficient. Look for students to identify using a vertical written algorithm, which involves renaming, as "less efficient", and "adding on using an empty number line", which does not involve renaming, as a "more efficient" strategy.

14.
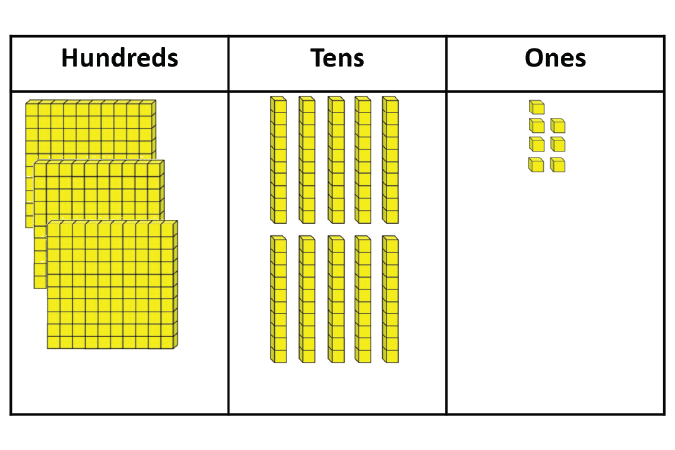
If appropriate, you could use this moment as a time to systematically teach the need to rename digits in the above sum, if it is represented using a vertical written algorithm. 806 is renamed as 7 hundred, 9 tens, and 16 ones. You might ask students to show how this works with place value people.
15.
Pose further difference (subtraction) problems with three-digit whole numbers to encourage the development of students' fluency. Provide a mix of problems in which addition and subtraction are favoured. Examples might be:
- There are 613 students at Rere School and 297 students at Kopu School. How many more students are at Rere School than Kopu School?
- There are 784 students at Rere School and 320 students at Kopu School. How many more students are at Rere School than Kopu School?
- There are 638 students at Rere School and 271 students at Kopu School. How many more students are at Rere School than Kopu School?
1.
Provide further problems and encourage students to use calculators to estimate their answers. For example:
- There are 638 students at Rere School and 271 students at Kopu School. How many more students are at Rere School than Kopu School?
- Which answer is closest? 420, 400, 380, 360, 340, 320
- How do you know?
- Check with a calculator.
2.
Discuss estimation strategies.
- What about the numbers, which meant that the answer must be less than 400?
- How can we use 38 and 71 to get a more accurate estimate?
3.
Extend the difference problem to include four-digit whole numbers. Note that mental strategies and adding on are likely to become less efficient and more taxing on students' cognitive resources as digit size increases. Conversely, subtraction is likely to become more efficient as number size increases.
The quality of the images on this page may vary depending on the device you are using.