Tumble time
This is a level 2 geometry activity from the Figure It Out series. A PDF of the student activity is included.

About this resource
Figure It Out is a series of 80 books published between 1999 and 2009 to support teaching and learning in New Zealand classrooms.
This resource provides the teachers' notes and answers for one activity from the Figure It Out series. A printable PDF of the student activity can be downloaded from the materials that come with this resource.
Specific learning outcomes:
Predict rotation patterns.
Tumble time
Achievement objectives
GM2-7: Predict and communicate the results of translations, reflections, and rotations on plane shapes.
Required materials
- Figure It Out, Levels 2–3, Geometry, "Tumble time", page 22
- cardboard (optional)
- scissors (optional)
See Materials that come with this resource to download:
- Tumble time activity (.pdf)
Activity
Activity 1
The aim of the activity is to have students visualise the movement of well-known shapes and get them to focus particularly on the path (locus) of a point or area at a fixed position on the shape.
For example, the path of the point on the cube-shaped block in diagram a would be:

Encourage the students to attempt to draw the path of the marked point or area following successive quarter-turns in the case of quadrilaterals and third-turns in the case of the equilateral triangle.
Students can confirm their answers by making a two-dimensional model of each block from the card and turning it along a ruler edge (as shown below).
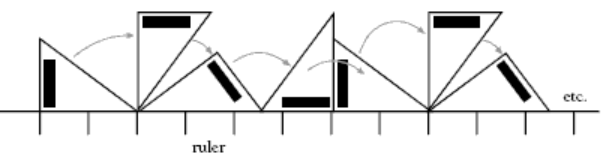
Another way to find the path of a point is to bore a small hole in the card and use the end of a pencil to trace the curve as the whole shape is turned.
Activity 2
The curved path traced by a point on the outer edge (circumference) of a circle as it turns is called a cycloid. Again, students can model this curve by cutting a circle from a card and tracing the path of the point through a small hole. Encourage them to sketch what they think the curve will look like before experimenting:
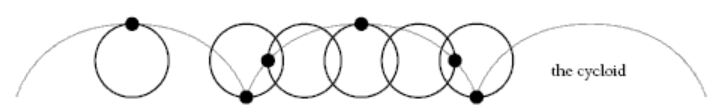
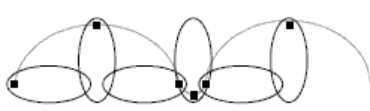
The curve traced by a point on the circumference of an ellipse has similar characteristics, though the height of the curve depends on the location of the point. Once again, this characteristic is easily explored with a cardboard ellipse (see the diagram in the answers section).
Activity 1
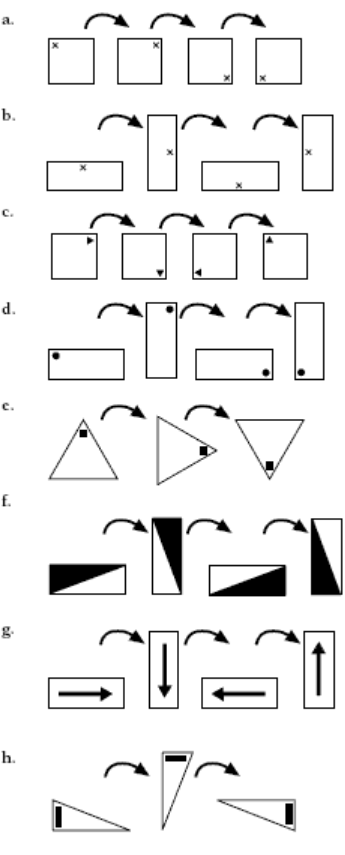
Activity 2
1.
a. It moves in a circular path as the circle turns. (It stays in the same position in relation to the outside of the circle.)
b.
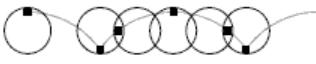
2.
a. The square in the ellipse will also move in a circular path (because the square is always the same distance from the centre of the ellipse).
b.
The quality of the images on this page may vary depending on the device you are using.


